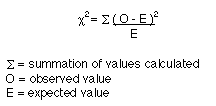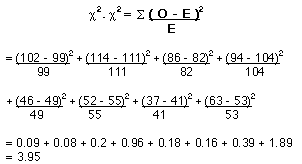In most of the Monarch’s breeding areas, there are four to fifteen species of milkweeds, all of which are potential hosts for Monarch larvae. These plants differ in many aspects, such as growth rates, toughness, hairiness, chemical make-up, and so on. This observation leads us to an interesting question about these varied plants: are they all equally attractive to Monarchs for oviposition and feeding? Let's examine the oviposition question. How could we determine if females prefer one species over another? We should start with a hypothesis. The best approach is to start with a null hypothesis (sometimes written H_o). The null hypothesis states that there is no difference between milkweed species A and B. The purpose of designing a test around a null hypothesis is to avoid the problem of unconsciously letting our expectations become part of our results. Our objective should be not to find that A is preferred more than B, if this is our expectation, but to establish in a neutral and objective way the preference or lack of preference shown by the butterflies. In other words, if we have prior knowledge, or think we do, that species A is preferred to species B, we might unintentionally obtain results which support this hypothesis. By suppressing our expectations, and doing a neutral test, we can let the butterflies tell us whether or not they show preferences. How could we conduct such a test?

In the field it is very difficult to follow Monarchs and keep track of which milkweed species they use for egg laying. Even if we made such observations, it might not be possible to tell which species they prefer. We would need to be able to determine if the ratio of contacts with the plants to eggs laid differed between or among milkweed species. However, in the laboratory we can provide a choice. To test for oviposition preference, we will need mated female Monarchs (how many?), a large flight cage, artificial nectar, and at least two species of milkweed which we can use to test for oviposition. If you set up such a cage and put in one species of milkweed and record the number of eggs per hour, you will notice that most of the egg laying occurs in the first 4-5 hours of light each day. From this observation, it becomes clear that if we wish to test for preference, we should do so in the morning hours.

To conduct a test
What must we control for when we compare milkweed species? To start, milkweeds selected for this test should be of similar condition, i.e., both have new leaves (it would be inappropriate to compare a plant of one species with old leaves to another with new leaves); both either with or without flowers (flowers may provide some type of stimulus - in fact, this is something we can test next: do plants with flowers within the same species receive more or fewer eggs than those without flowers); both should be the same size; and so on. Should we control the position of plants within the flight cages? There could be slight differences in the cage that could cause more eggs to be laid on the plant placed on one side of the cage than the other. Maybe the procedure should involve reversing the positions of the plants; if we do this, how many times should we do so, and how many replicates do we need (i.e., how many pairs of plants should we compare)? Will two be enough? Four better? And how long should the test interval be? 10 minutes? 20 minutes? 30 minutes? Should we use new plants on each test for each replicate? A few preliminary tests could be conducted to answer these procedural questions.

Analyzing the results
After we have counted the eggs on each plant in each replicate, we can analyze the data to determine if there are differences between these plants in attractiveness. The choice of the approach here depends on the level of sophistication of the students we are working with. We could simply total the results and if there were no substantial difference in the number of eggs laid on A and B, it would be clear that the butterfly treats both species in a similar manner. If the students can handle relatively simple statistics, a chi-squared test or a t-test could be performed. These are described in any beginning statistics book.

Interpretation of results
What are the possible interpretations of the results? The students can conclude either that: (1) there is no difference between A and B, or (2) there is a difference between A and B. Should the results indicate a difference between these or other species, the task then becomes how to interpret this difference. Are there differences between the two species which are apparent to us? If the students notice that A and B differ in leaf texture or hairiness, they might hypothesize that this may influence oviposition preference. Could the butterflies be using the same senses we do to select plants? Or, do the butterflies use chemical information not available to us. If so, how do they perceive these signals and what types of chemicals are likely to be involved? Is there a way we could make a less attractive species more attractive? By asking these questions the students are forced to make additional observations which can lead to questions and other hypotheses to test.

Alternatives
If you don't have more than one species of milkweed, but you have plants with flowers and plants with no flowers (remember, you can always cut flowers off of flowering stalks), you could conduct tests to determine if the Monarchs show a difference in egg laying in the presence or absence of flowers. Similarly, we could ask whether females prefer to lay eggs on young (new) or old leaves. If you can reject the null hypothesis, and there are more eggs laid on plants with or without flowers or new vs. old leaves, the students should be led to hypothesize why. This could lead to more experiments, for example using filter paper extract of flowers, leaves, or both to help determine causes for these differences.

Add ons
Access to newly emerged Monarchs, milkweed plants and cages could be used by students to make observations on mating behavior, e.g., age at first mating, number of matings, time of day mating occurs, etc. In addition, students could observe and record details about egg laying (oviposition) behavior, e.g. time of day, intervals between each oviposition, choice of oviposition sites, number of eggs per day, and number of eggs per lifetime. These data could be graphed in a number of different ways to show lifetime patterns and differences between individuals. If students make observations of egg laying they will notice that females lay most of their eggs on the underside of leaves. This leads to a “why” question that could be used to discuss the advantages and disadvantages of placing eggs on upper vs. lower surfaces of leaves, and this discussion could lead to another experiment. What happens when we turn the plants upside down? Will the females continue to lay eggs on the lower surface (now upper surface) as they did before? To conduct this test the students will have to figure out a way of suspending the plants upside down (without making a mess of everything). One possible method is to use stem holders for roses which are available at most florists.

If students carry out the basic experiment outlined above and obtain results which indicate that females lay significantly more eggs on one type of plant, the next question is what is the underlying basis for the difference? Do the females make choices before they land on the plants or after they have made contact? This question could be examined by making careful observations and records of ratios of contact to egg laying.

Remember, to facilitate observations and data collecting, Monarchs can be marked to distinguish one from another - either with the use of tags or by using a permanent marking pen to give each individual a number in the discal cell on the underside of the hindwing.

EXAMPLE OUTCOMES

Number of eggs laid by female Monarchs (N=20) in 15 minute intervals.
(R) = plant positioned on right side of cage; (L) = plant positioned on left side of cage.

Species A Species B Trial 1 102 (L) 46 (R) Trial 2 52 (R) 114 (L) Trial 3 86 (L) 37 (R) Trial 4 63 (R) 94 (L)

Is there a difference between A and B? Is there a position effect in the cage?

Various statistical tests can be used to ask if there is a significant difference between two observations or sets of outcomes. The Chi Square (X²) test is frequently used to determine whether differences are likely to be due to chance, referred to as sampling variability (or sampling error), or likely to occur so rarely that they represent real or significant differences between two data sets. If the differences between A and B are so small as to be due to chance, then there is no significant difference between them and we are unable to reject the null hypothesis. If, on the other hand, the difference between A and B is significant at a probability of <.05% then we can reject the null hypothesis.

Steps for Performing X² Statistics

1) State the Null Hypothesis (H₀) For example, female Monarchs show no egg-laying preferences and will lay similar numbers of eggs on milkweed species A and milkweed species B.

2) Calculate ECF (Expected Cell Frequency) based on marginal frequencies from the observed data.

Note: The expected data table with ECFs calculated.

3) Fill the expected data table with ECFs calculated.

4) Calculate X². The X² formula compares expected and observed cell frequencies to measure the difference between observed and expected values.

5) Calculate degrees of freedom (d.f.)

d.f. =( r-1)(c-1)

r = number of rows
c = number of columns

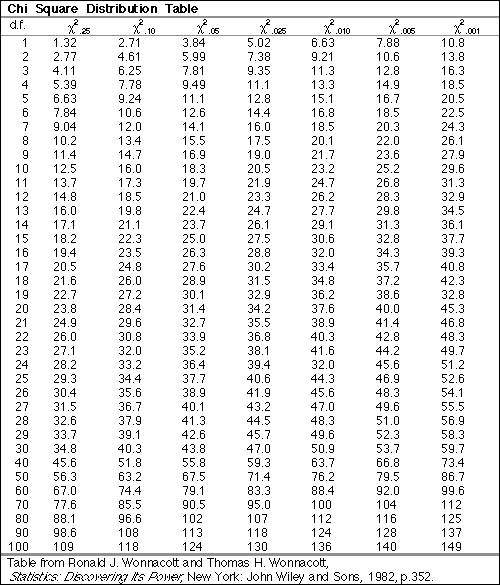
Degrees of freedom is the number of quantities that are unknown minus the number of independent equations linking these unknowns. For a contingency table, this is the number of cells that must be filled in to determine all the cells. For example: a two by two table, the row and column marginals are known and the value in only one cell needs to be calculated to figure out all the other cells. i.e. d.f. = (2-1)(2-1)= 1

6) Select a significance level. Most often .05 level is chosen. (Significance at the .05 level means that 5 out of 100 times the differences observed may have occurred by chance).

7) Look up the X² value on the Chi Square Distribution Table (below). Look at the value under the chosen significance level and the degrees of freedom calculated.

8) Check to see if the computed X² value is greater than the table value.

If larger, then H₀ is rejected and there is a significant difference.
If smaller, then failed to reject H₀ and there is no significant difference.

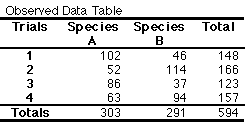
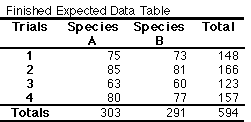
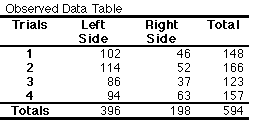
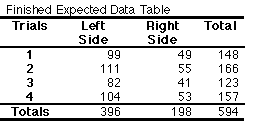
EXAMPLES USING THE GIVEN OBSERVED NUMBERS

EXAMPLE 1: Is there an egg-laying preference between the two species of milkweed by the Monarch?

1) State the Null Hypothesis: There is no difference in egg laying preference between milkweed species A and B.

2) Calculate ECF, and 3) Fill the Expected Data Table

The values bolded are the marginal frequencies from the observed data (or known values).

Calculate the ECF for Species A - Trial 1 = (148 x 303) / 594
ECF = 75

Calculate the rest of the ECF’s for Species A. Then finish filling in the table by simple subtraction from the totals for each row.

4) Calculate

5.) Calculate d.f. (4 - 1)(2 - 1) = 3

6) Significance level at .05

7) X² value in table = 7.81

8) Compare the computed X² and the X² table value.

The computed value is larger, therefore the H₀ is rejected. The relationship between milkweed species A and B is statistically significant.

EXAMPLE 2: Is there a position effect in the cage?

1) State the Null Hypothesis: There is no difference in preference of the plant placed on the left side or right side of the cage for egg laying.

2) Calculate ECF, and 3.) Fill the Expected Data Table

Calculate the ECF for Left Side - Trial 1 = (396 x 148) / 594
ECF = 99

Calculate the rest of the ECF’s for the Left Side. Then finish filling in the table by simple subtraction from the totals for each row.

4) Calculate

5) Calculate d.f. (4 - 1)(2 - 1) = 3

6) Significance level at .05

7) X² value in table = 7.81

8) Compare the computed X² and the X² table value.

The computed value is smaller, therefore failed to reject the H₀. The there is no position effect of the plant placed on the left or the right side of the cage.

Chi Square Distribution Table

References:

M. C. Fleming and J. G. Nellis, Principles of Applied Statistics, New York: Routledge, 1994, p. 400.

A. D. Rickmers and H. N. Todd, Statistics: An Introduction, St. Louis: McGraw-Hill, 1967, p. 585.